Graphs are used to represent solving complex relationships and dependencies in various fields such as machine learning and AI. They are ideal for modeling systems like social networks, communication networks, and transportation systems. In this article, we’ll explore the components, types, and practical applications of graphs, as well as their role in specialized use cases and real-life scenarios.
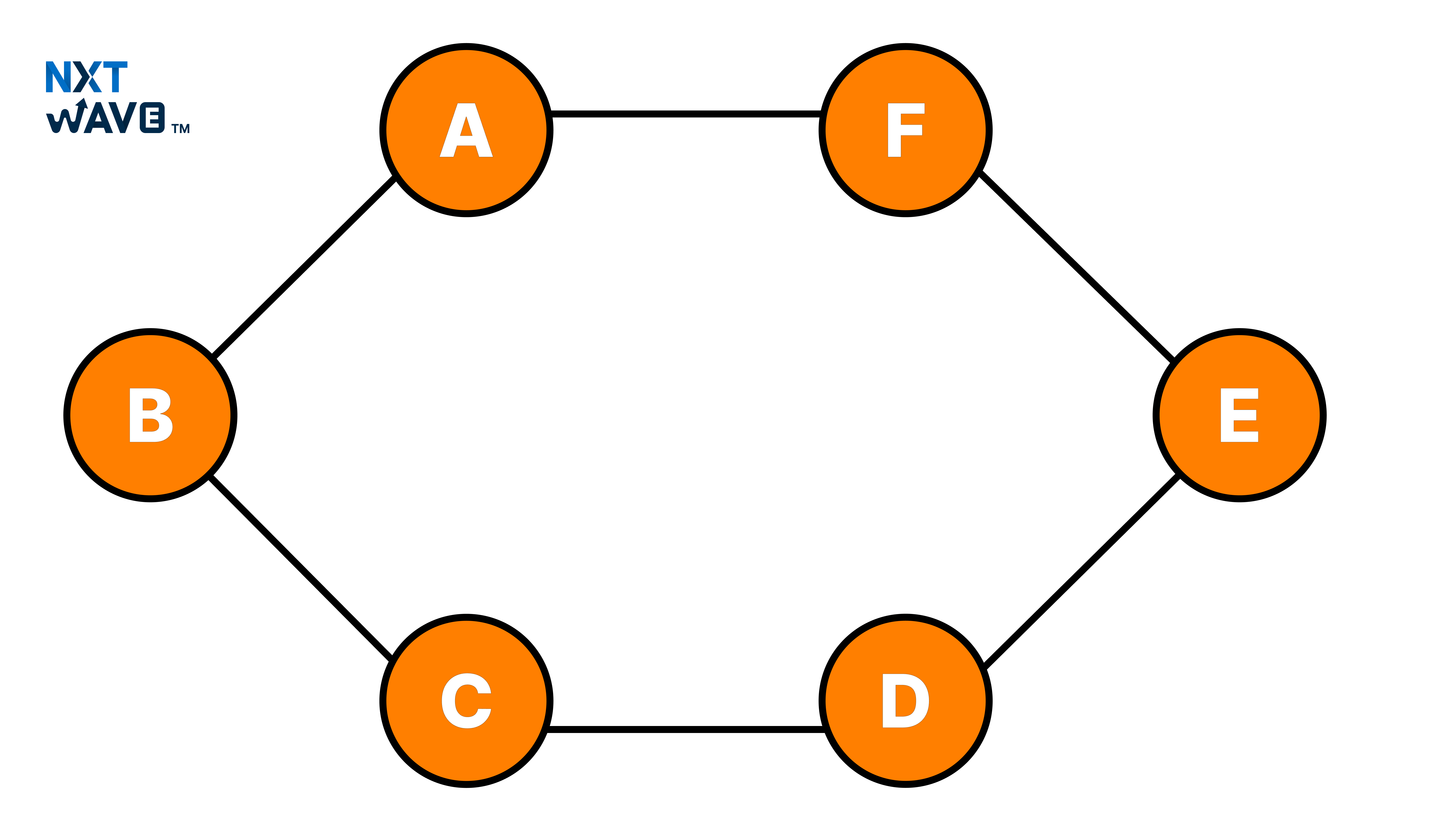
A graph is a data structure that consists of a set of vertices (or nodes) and a set of edges (or arcs) that connect pairs of vertices. It is used to model relationships or connections between objects.
It can be defined as G = (V, E), where:
A graph consists of two primary components:
Here are the types of graphs in data structures:
A graph with no vertices and no edges. It is the most basic form of a graph, where there are no connections between any nodes.
A graph with exactly one vertex and no edges. It is a special case of a graph with the smallest possible number of vertices.
A graph where the edges have no direction. In other words, if there is an edge between vertex A and vertex B, it means A is connected to B, and B is also connected to A.
A graph in which edges have a direction. Each edge is represented as an ordered pair of vertices, where the edge goes from one vertex (the source) to another (the destination).
A graph in which there is a path between every pair of vertices. All vertices are reachable from any other vertex in the graph.
A graph where some vertices are not connected to others. There are at least two disconnected components.
A graph that forms a closed loop, meaning all vertices are connected in a single cycle without any additional edges or vertices.
A graph that contains at least one cycle (a closed path where the starting and ending vertex are the same). Not all cyclic graphs are cycle graphs.
A graph that does not contain any cycles. In other words, there is no closed path in the graph.
A graph where every vertex has the same number of edges (degree). A regular graph can be k-regular if every vertex has exactly k edges.
A graph where there is an edge between every pair of vertices. In a complete graph with
n vertices, each vertex is connected to n−1 other vertices.
The vertices and edges of a graph are finite.
A graph with an infinite number of vertices and/or edges.
A graph where the vertices can be divided into two disjoint sets such that every edge connects a vertex in one set to a vertex in the other set. No edge exists between vertices within the same set.
The graph consists of no loops (edges connecting vertices together) or multiple edges between vertices.
It is a graph in which two vertices can have multiple edges connecting them. In other words, there can be more than one edge connecting the same pair of vertices.
A labeled graph is a graph where each vertex and/or edge is assigned a label or identifier. These labels could represent unique names or properties associated with vertices or edges.
A subgraph is a subset of a graph, where some vertices and edges of the original graph are selected. A subgraph must include all edges whose endpoints are in the selected vertices.
A pseudograph is a graph that allows both multiple edges (parallel edges) between the same pair of vertices and self-loops (edges that connect a vertex to itself).
A planar graph is a graph that can be drawn on a plane with its edges. In other words, there exists a way to draw the graph such that two edges intersect each other.
The Euler graph in which there exists a closed trail (Eulerian circuit) that visits every edge exactly once. A graph is Eulerian if all of its vertices have an even degree (the number of edges incident to the vertex).
A graph that contains a Hamiltonian cycle that visits each vertex exactly once and returns to the starting vertex. A graph is Hamiltonian if there exists a cycle that passes through every vertex once and only once.
Graphs have numerous applications in both theoretical and practical computing. Here are some of the them as listed below:
Graphs play a crucial role in Machine Learning and AI:
Here are the advantages of graphs in data structures:
In conclusion, graphs are high with wide-ranging applications in both theoretical and practical domains. An application graph in data structure refers to the outcome or result of using a graph data structure to model and solve a real-world problem. It is the final stage of applying graph theory to a specific domain or application, where the graph is used to represent relationships, connections, or patterns between entities.
Graphs are used in many real-life applications like social networks (representing people and their connections), routing algorithms (for navigation), and recommendation systems (like those used by Amazon or Netflix).
Specialized graphs include bipartite graphs, which are used for matching problems, and tree graphs, which are used in hierarchical structures. Planar graphs are used in geographical mapping, and dependency graphs are used in project management.
